题目大意
根据二叉树的前序遍历和中序遍历( 中序和后序)结果生成二叉树
假设没有重复数字
解题思路
参考给中序和后序遍历
看到树首先想到要用递归来解题。以这道题为例:如果一颗二叉树为{1,2,3,4,5,6,7},则中序遍历为{4,2,5,1,6,3,7},后序遍历为{4,5,2,6,7,3,1},我们可以反推回去。由于后序遍历的最后一个节点就是树的根。也就是root=1,然后我们在中序遍历中搜索1,可以看到中序遍历的第四个数是1,也就是root。根据中序遍历的定义,1左边的数{4,2,5}就是左子树的中序遍历,1右边的数{6,3,7}就是右子树的中序遍历。而对于后序遍历来讲,一定是先后序遍历完左子树,再后序遍历完右子树,最后遍历根。于是可以推出:{4,5,2}就是左子树的后序遍历,{6,3,7}就是右子树的后序遍历。而我们已经知道{4,2,5}就是左子树的中序遍历,{6,3,7}就是右子树的中序遍历。再进行递归就可以解决问题了。
前序和中序:
1
2
| root.left = self.buildTree(preorder[1 : index + 1], inorder[0 : index])
root.right = self.buildTree(preorder[index + 1 : len(preorder)], inorder[index + 1 : len(inorder)])
|
中序和后序:
1
2
| root.left = self.buildTree(inorder[0 : index], postorder[0 : index])
root.right = self.buildTree(inorder[index + 1 : len(preorder)], postorder[index : len(inorder)-1])
|
代码
前序和中序
1
2
3
4
5
6
7
8
9
10
11
| class Solution(object):
def buildTree(self, preorder, inorder):
if len(preorder) == 0:
return None
if len(preorder) == 1:
return TreeNode(preorder[0])
root = TreeNode(preorder[0])
index = inorder.index(root.val) # 中序中根节点的位置,左边即为左子树,右边由子树
root.left = self.buildTree(preorder[1 : index + 1], inorder[0 : index])
root.right = self.buildTree(preorder[index + 1 : len(preorder)], inorder[index + 1 : len(inorder)])
return root
|
中序和后序
1
2
3
4
5
6
7
8
9
10
11
| class Solution(object):
def buildTree(self, inorder, postorder):
if len(inorder) == 0:
return None
if len(inorder) == 1:
return TreeNode(inorder[0])
root = TreeNode(postorder[len(postorder) - 1])
index = inorder.index(postorder[len(postorder) - 1])
root.left = self.buildTree(inorder[ 0 : index ], postorder[ 0 : index ])
root.right = self.buildTree(inorder[ index + 1 : len(inorder) ], postorder[ index : len(postorder) - 1 ])
return root
|
总结
二叉树遍历
二叉树的前序、中序、后序遍历(深度优先遍历)
遍历即将树的所有结点都访问且仅访问一次。按照根结点访问次序的不同,可以分为前序遍历,中序遍历,后序遍历。
前序遍历:根结点 -> 左子树 -> 右子树
中序遍历:左子树 -> 根结点 -> 右子树
后序遍历:左子树 -> 右子树 -> 根结点
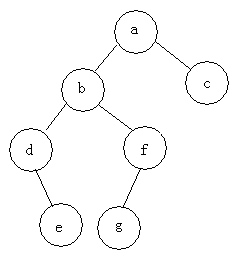
前序遍历:abdefgc
中序遍历:debgfac
后序遍历:edgfbca
层次遍历(广度优先遍历)
层次遍历:abcdfeg
